Stability-endangering vibration

Structures are designed to withstand static loads, i.e. loads that are constant over time. This means that the entire structure and its load-bearing components must be dimensioned in such a way that they can transfer the loads from these actions and thus remain stable. One static load that every structure must bear is its own weight.

In addition to the static, i.e. time-independent loads, there are also a number of time-dependent loads that act on structures. These dynamic loads must also be transferred by the building's supporting structure.

Such dynamic loads arise, for example, from

• Traffic
• Machine operation
• Human movements
• Wind
• Waves
• Impact
• Explosion
• Earthquake

The range of structures that are designed to withstand dynamic loads extends from engineering structures such as bridges, towers, tunnels and high-rise buildings to structural facilities for energy generation and/or high-risk potential, such as nuclear power plants, chemical and wind power plants, and ordinary residential buildings.

Strictly speaking, all conceivable loads acting on a structure are time-dependent, i.e. dynamic. Even the dead weight is applied at a certain point in time (that of the manufacture of the component) and is therefore time dependent. The essential difference to the dynamic loads mentioned above is that the effects of mass inertia can be neglected in the case of the so-called static loads. In the case of dynamic loads, on the other hand, the effects of mass inertia and damping play the decisive role.

The timing or exact location of the occurrence of a dynamic load variable is often unknown or variable. For example, in the case of a ceiling in a residential building, it is not known in advance how many people will cross it, which path they will take, at what speed they will walk, whether they will stop or bounce, etc.. But even for much larger loads, such as earthquakes or loads from airplane crashes, an enveloping consideration is often made over several possible scenarios from a probabilistic point of view. For example, the load assumptions of a standard earthquake design cover a large number of possible earthquakes. Therefore, the dynamic load specifications are usually to be understood as an envelope of several dynamic load cases.

Dynamic loads are only partially actually considered as time-dependent in the design of structures. Instead, simplified static equivalent loads are often used for the design of structures. For this purpose, the dynamic loads acting on a building structure must be converted into equivalent static loads.

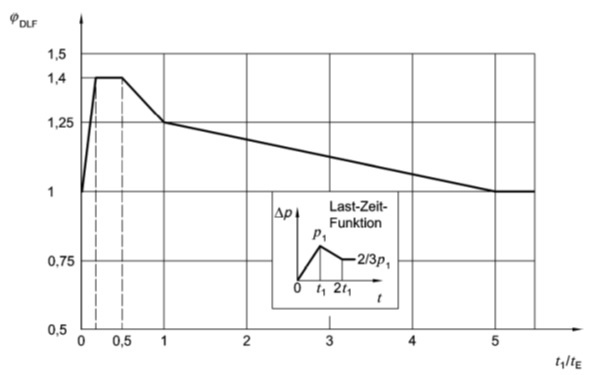
However, by considering only the maximum value of the time-varying load, the dynamic behavior, i.e. the effects of inertia, damping, etc., is not adequately captured. Due to the dynamic effects mentioned above, the static load equivalent to the dynamic load can be increased or reduced. For an adequate consideration of the behavior of a structure loaded by a dynamic effect, it is therefore not sufficient to use the maximum value of the temporal course of the load as the static load. Rather, it is necessary to multiply this maximum value by a so-called dynamic load factor. This dynamic load factor contains the dynamic excitation character of an individual load depending on the vibration behavior of the loaded building structure.

The figure shows an example of the dynamic load factor as a function of the vibration period for an Explosions pressure wave load.

It is therefore important that not only the time-dependent course of the load but also the vibration behavior of the loaded structure plays a role in the dynamic design of building structures. The relationship between the excitation frequency of the load and the response frequency of the loaded structure is of decisive importance. If the two frequencies are close to each other, a so-called resonance effect occurs, which can increase the load on the structure by a multiple of the maximum value of the load.

Knowledge of the main frequencies of the excitation is therefore also a prerequisite for modeling the system loaded by the dynamic action. At low excitation frequencies, such as earthquakes or wind, whole buildings can be vibrated, while at high-frequency dynamic loads, for example impact loads, local phenomena in the loaded component are additionally important.

The interaction of dynamic excitation and dynamic response of the building structure must therefore be considered in the model representation. Depending on the phenomenon of interest, different models may be used for the same load case. For example, if an airplane hits a reinforced concrete wall, highly dynamic effects occur in a locally confined area. If a component is to be designed for the area directly hit, a detailed model of this local area is required. If, on the other hand, the stability of the entire building is to be ensured, phenomena in the lower frequency range play a role and a high level of detail of the impact location is not necessary. Instead, the essential dynamic properties of the entire building must be modeled.